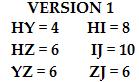Unit 5: Geometry and Measurement
Topic 1: Triangle Similarity Theorems
Competencies
·
State
similarity triangle theorems
·
Apply
similarity triangle theorems
·
Apply
similarity ratio on similar triangles
Suggested ways of teaching this topic: Guided practice,
Q&A, small group discussion,…
Starter Activities
The teacher may start with the brainstorming
question like:
·
Who can tell me
the definition of similar triangles? Or
·
When do we say
two triangles are similar?
·
What kind of
symbol do we use to denote similarity?
·
Who can
demonstrate the similarity of two triangles in drawing?
Expected Answer
Triangles
are similar if their corresponding (matching) angles are congruent (equal) and
the corresponding sides are in same proportion.
 The Symbol for
Similar is:
The Symbol for
Similar is:![]()
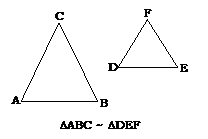
When
we say DABC
~ DDEF,
- The 3
corresponding angles are congruent
i.e. ÐA ºÐD, ÐB ºÐE, ÐC ºÐF
- The 3
corresponding sides are in proportion
i.e. ![]()
The
teacher shall ask the following question to make sure students understood the
idea of proportional sides in similar triangles.
·
If
DABC ~ DXYZ then list
down as many proportional equations as you can.
Expected Answer:
DABC ~ DXYZ Þ![]()
DABC ~ DXYZ Þ![]()
DABC
~ DXYZ Þ![]()
DABC
~ DXYZ Þ![]()
The
similarity ratio is the proportion that you get when you divide any
corresponding side of one triangle by its corresponding side in other similar
triangle.
Lesson
Notes
 The teacher
shall use the following examples to let students have a better practice on
finding ratio of sides in similar triangles.
The teacher
shall use the following examples to let students have a better practice on
finding ratio of sides in similar triangles.
Example: Let DABC ~ DEFD as shown in
the figure. Find the length of ![]()
Solution: DABC ~ DEFD Þ![]()
 Þ
Þ![]() Þ ED =
Þ ED = ![]() = 16cm
= 16cm
DABC ~ DEFD Þ![]() Þ
Þ![]()
Þ![]() Þ FD =
Þ FD = ![]() = 20cm
= 20cm
Example: In the figure,
if DABC
~ DADE,
What is the length of AC?
Solution: DABC ~ DADE Þ![]()
Þ![]()
EC
= 6.4 – 4 = 2.4cm
AC
= AE + EC
=
4 + 2.4 = 6.4cm
The
teacher should ask students about the relationship between congruent and
similar triangles. The following example might help.
Example: Prove that if DABC ºDDEF, then DABC ~ DDEF
Proof: DABC ºDDEF Þ AB = DE, BC =
EF and AC = DF
Þ![]() =1
=1
That
is, if two triangles are congruent then they are similar.
Teacher
can ask students: “Is the converse statement true?”
Expected
Answer
 In similar
triangles, only the angles are congruent but the sides are not necessarily
congruent. Therefore, similar triangles may not be congruent.
In similar
triangles, only the angles are congruent but the sides are not necessarily
congruent. Therefore, similar triangles may not be congruent.
Property
of a line parallel to a side of a triangle
If a
line parallel to a side of a triangle is drawn between the side and the
opposite angle, then it divides the other two sides proportionally
That
is, ![]() or
or ![]()
After a
thorough discussion on the similarity ratio, the teacher may guide students
with the following similarity theorems, their proofs and applications.
The AAA Theorem
If all
the three angles of DABC is congruent to the
corresponding angles of DDEF,
DABC ~ DDEF
Proof
Given: DABC and DDEF withÐA ºÐD, ÐB ºÐE and ÐC ºÐF
To
prove: DABC
~ DDEF
Assume
that AC > DF and BC > EF and mark points P and Q on ![]() such that PC =
DF and QC = EF
such that PC =
DF and QC = EF
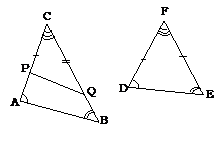
Statement Reason
1.
![]() 1. Construction
1. Construction
2.
ÐC
ºÐF 2. Given
3.
![]() 3.
Construction
3.
Construction
4. DPCQ ºDDFE 4. SAS postulate
5. ÐCPQºÐD and ÐCQPºE 5. Corresponding angles of congruent
triangles
6. ÐCPQºÐA and ÐCQPºÐB 6. Transitivity of congruence of angles
7.
![]() 7.
Corresponding sides of congruent triangles
7.
Corresponding sides of congruent triangles
8.
![]() 8. Property of a line
parallel to side of a triangle
8. Property of a line
parallel to side of a triangle
9.
![]() 9.
Substitution
9.
Substitution
Similarly,
![]()
 Since the
corresponding angles are congruent and the corresponding sides are
proportional, we have DABC ~ DDEF.
Since the
corresponding angles are congruent and the corresponding sides are
proportional, we have DABC ~ DDEF.
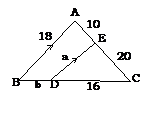
Example: In the figure,
if ![]() Calculate a, b and c
Calculate a, b and c
Solution: ∆ECD
≡ ∆ACB by AAA
Þ![]()
Þ![]()
![]()
Theorem: (AA theorem)
If two
angles of one triangle are congruent to two angles of another triangle, then
the triangles are similar.
The teacher could leave the proof to the
students giving the clue that they can use the previous AAA Theorem.(Of course, they shall use angle sum theorem of triangles!)
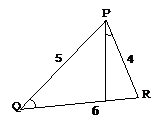 Example: In the figure, if PQ = 5, QR = 6, PR = 4
and ÐPQR
ºÐRPK, find KR and KP.
Example: In the figure, if PQ = 5, QR = 6, PR = 4
and ÐPQR
ºÐRPK, find KR and KP.
Solution:
Statement Reason
1. ÐR ºÐR 1. Common angle
2.
ÐPQR
ºÐRPK 2. Given
3. DRQP ~ DRPK 3. AA similarity theorem
Þ![]()
Þ![]()
The SAS Similarity Theorem
If two
sides of a triangle are proportional to two sides of another triangle and the
angle included in these sides are congruent, then the triangles are similar.
 Proof:
Proof:
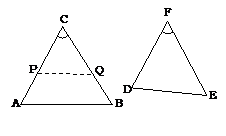
Given:DABC and DDEF with
ÐC º F, ![]()
To prove:DABC ~ DDEF
Construction:
Assume
AC > DF and BC > EF. Mark points P and Q on ![]() and
and ![]() respectively such that
respectively such that ![]() and
and ![]()
Statement Reason
1. ![]() 1.
Construction
1.
Construction
2. ÐC ºÐF 2. Given
3. ![]() 3.
Construction
3.
Construction
4.
DPQC
ºDDEF 4. SAS postulate
5. ![]() 5.
Given
5.
Given
6. ![]() 6.
Substitution
6.
Substitution
7. ![]() 7.
property of propotional line
segments
7.
property of propotional line
segments
8. ÐCPQ ºÐA, ÐCQP ºÐB 8.
Corresponding angles
9. ÐD ºÐA, ÐE ºÐB 9. Substitution
10. DABC ~ DDEF 10. AA similarity theorem
Example: Are the given
pairs of triangles similar?
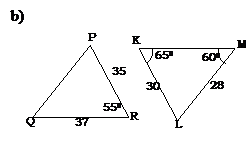 a)
a)

Solution:
A)
congruent
angles: ÐR ºÐL
Sides in proportion: ![]()
Therefore, DPQR ~ DNML since ÐR ºÐL and ![]()
B)
m(ÐL) = 1800
– (650 + 600) = 550 = m(ÐR)
![]()
Therefore,
DPQR
is not similar to DKLM
The SSS Similarity Theorem
If
three sides of one triangle are proportion to three sides of another triangle,
then the two triangles are similar.
Proof
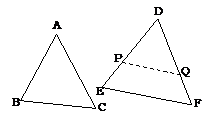 Given: DABC and DDEF, With
Given: DABC and DDEF, With ![]()
To
prove: DABC
~ DDEF
Construction: Assume that DE
> AB and DF > AC.
Make
point P on![]() such that
such that![]() . Then draw a line parallel to
. Then draw a line parallel to![]() meet DF at Q.
meet DF at Q.
Statement Reason
1.
ÐDPQ
ºÐDEF 1. Corresponding
angles
2. ÐD ºÐD 2. Common angle
3. DDPQ ~ DDEF 3. AA similarity theorem.
4. ![]() 4. Def. of similar
triangles.
4. Def. of similar
triangles.
5. ![]() 5. Given
5. Given
6. ![]() 6. Steps 4
& 5 and substitution
6. Steps 4
& 5 and substitution
7. DABC ºDDPQ 7. SSS theorem
8. DABC ~ DDEF 8. Step 3 and substitution
Concluding Activities
The teacher
shall make sure that the following are summarized by the students. The
applications of the theorems are also well practiced through teacher supervised
exercises.
§ Two triangles
are said to be similar if all their corresponding angles are congruent and the
corresponding sides are proportional.
§ The AA similarity theorem: If two angles of
one triangle are congruent to two angles of another triangle, then the
triangles are similar.
§ The SAS Similarity Theorem: If two sides of
a triangle are proportional to two sides of another triangle and the angle
included in these sides is congruent, then the triangles are similar.
§ The SSS Similarity Theorem: If three sides
of one triangle are proportional to three sides of another triangle, then the
two triangles are similar.
§ Congruent
triangles are similar but similar triangles may not be congruent.
Practice Exercises
|
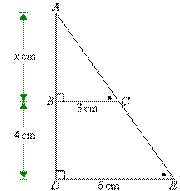
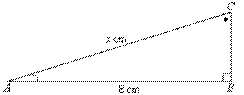
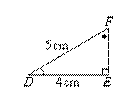
1. Find the value of x in the following pair of triangles.
|
|
|
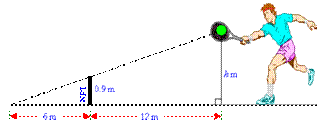
2. Find the value of the height, h m, in the following diagram at which the tennis ball must be hit so that it will just pass over the net and land 6 m away from the base of the net.
|
|
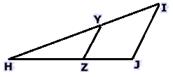
3. Below are two versions of ΔHIZ and ΔHYZ. Which of these two versions give a pair of similar triangles?
|
|
|
|
4.
In the figure, ΔTUV and ΔTWX are similar withTU = 10 and TW = 40,
what is the similarity ratio?

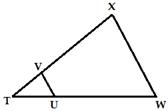
5. In the
following figure, ED // BC. A, B and E are collinear and A, C and D are also collinear.
Find AC and AB